Modular Origami
One of my hobbies is constructing various solids using modular origami.
The models below are mostly made of few to 150+ identical origami units. Theoretically, no cutting or gluing is required for the construction, but I sometimes use glue to make the bodies hold better and longer. The number of days that takes me to make a model is approximately the number of units divided by 3 (because on average I only do about 10 minutes of folding every day).
Some mathematical comments are spread throughout the models. Feel free to ignore them.
If you want to skip the comments and just look at pictures, you can also chek the gallery.
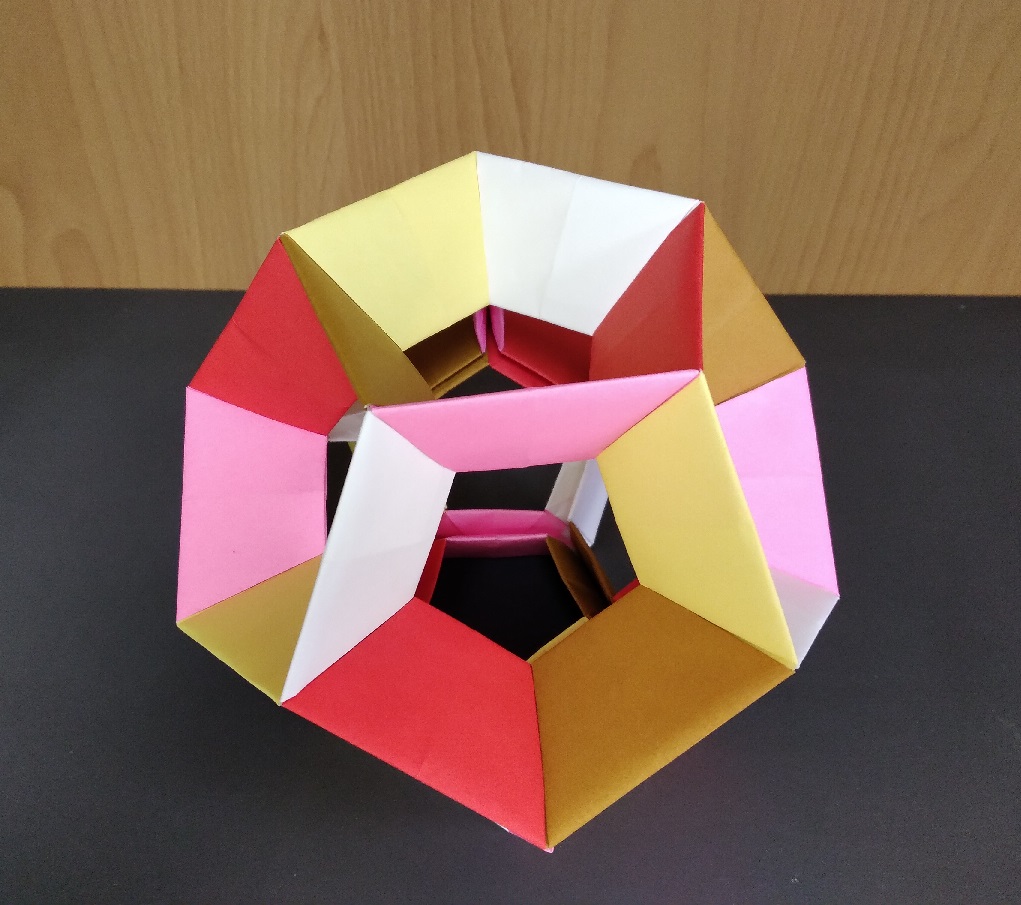
Dodecahedron
Constructed at University of Haifa, 2020.
Made of 30 identical pieces in 5 colors.
The symmetry group is A5 and the coloring consists of orbits of a subgroup isomorphic to A4.

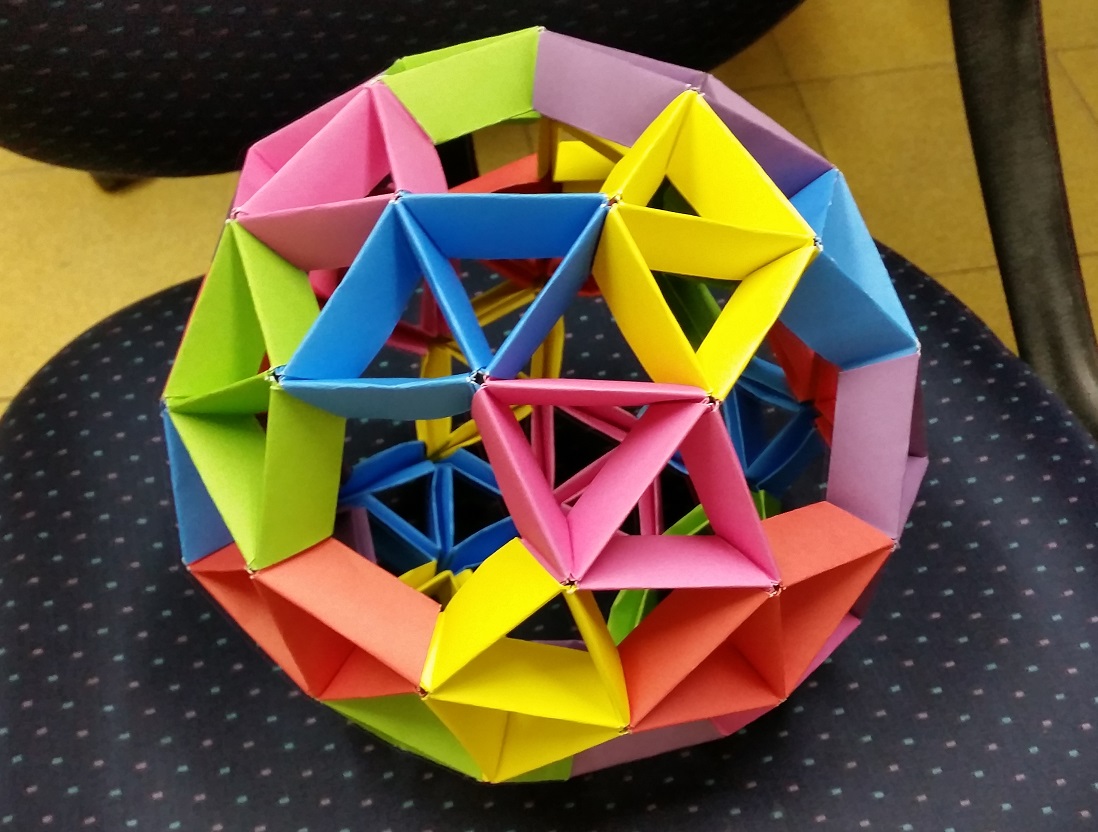
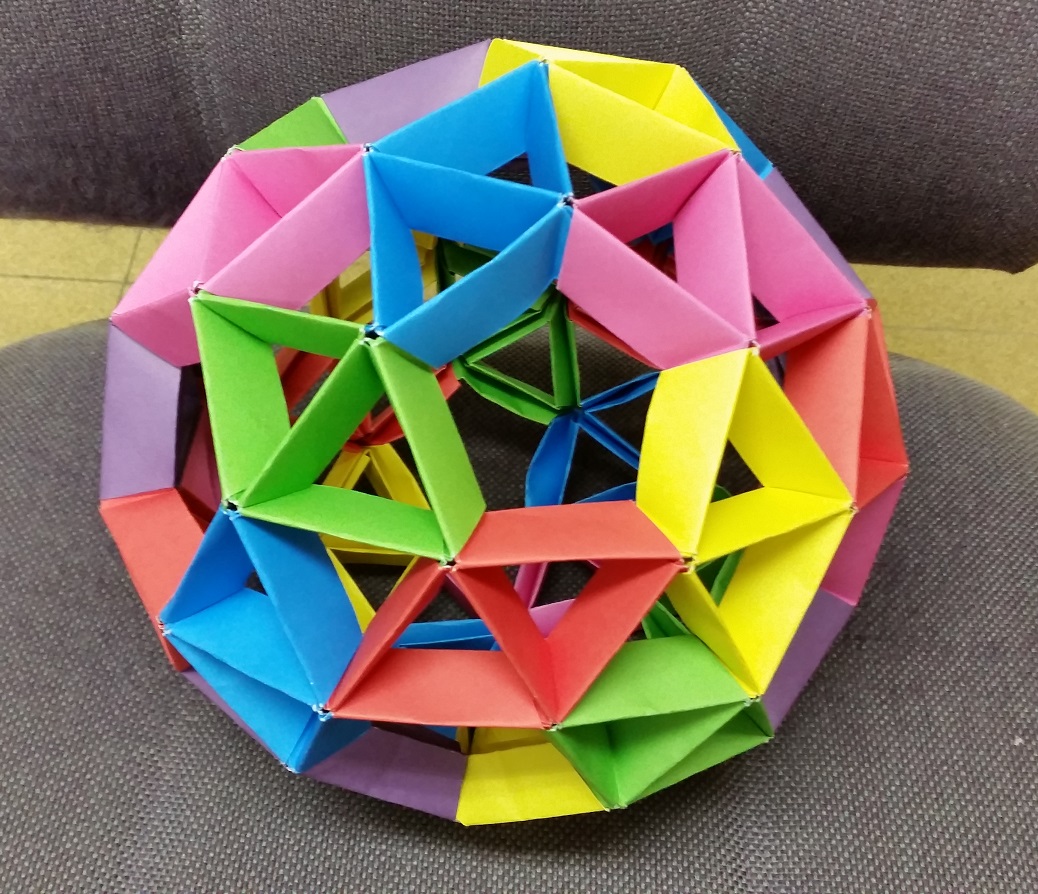
Snub Dodecahedron
Constructed at University of Haifa, 2019.
Made of 150 identical pieces in 6 colors.
The symmetry group is A5 and the coloring consists of orbits of a subgroup isomorphic to D10.
Cuboctahedron
Constructed at University of Haifa, 2017.
Made of 24 identical pieces in 4 colors.
The symmetry group is S4 and the coloring "proves" it: Any rotation of the solid
induces a permutation of the coloring (which consists of 4 colors). This map
induces an isomorphism of the symmetry group into the group of permutations on 4
colors.


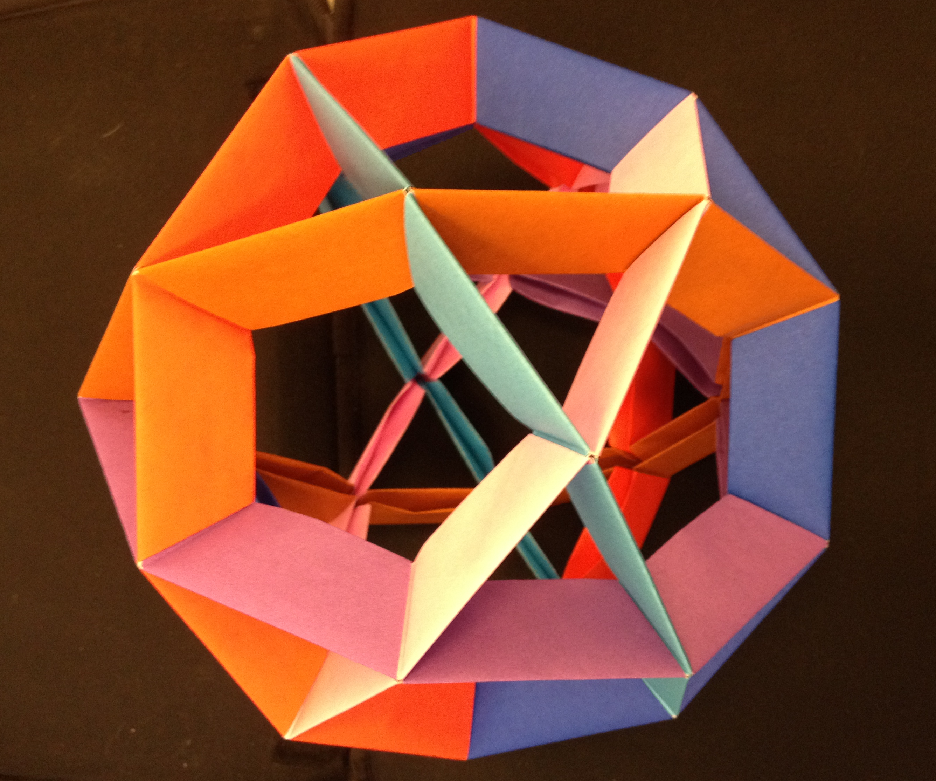
Icosidodecahedron (with Pyramids on the Faces)
Made of 60 identical pieces in 5 colors.
The symmetry group is A5 and the coloring "proves" it: Any rotation of the solid
induces a permutation of the coloring (which consists of 5 colors). This map
induces an isomorphism of the symmetry group into the group of even permutations on 5
colors.
For more information about icosidodecahedrons, see here.

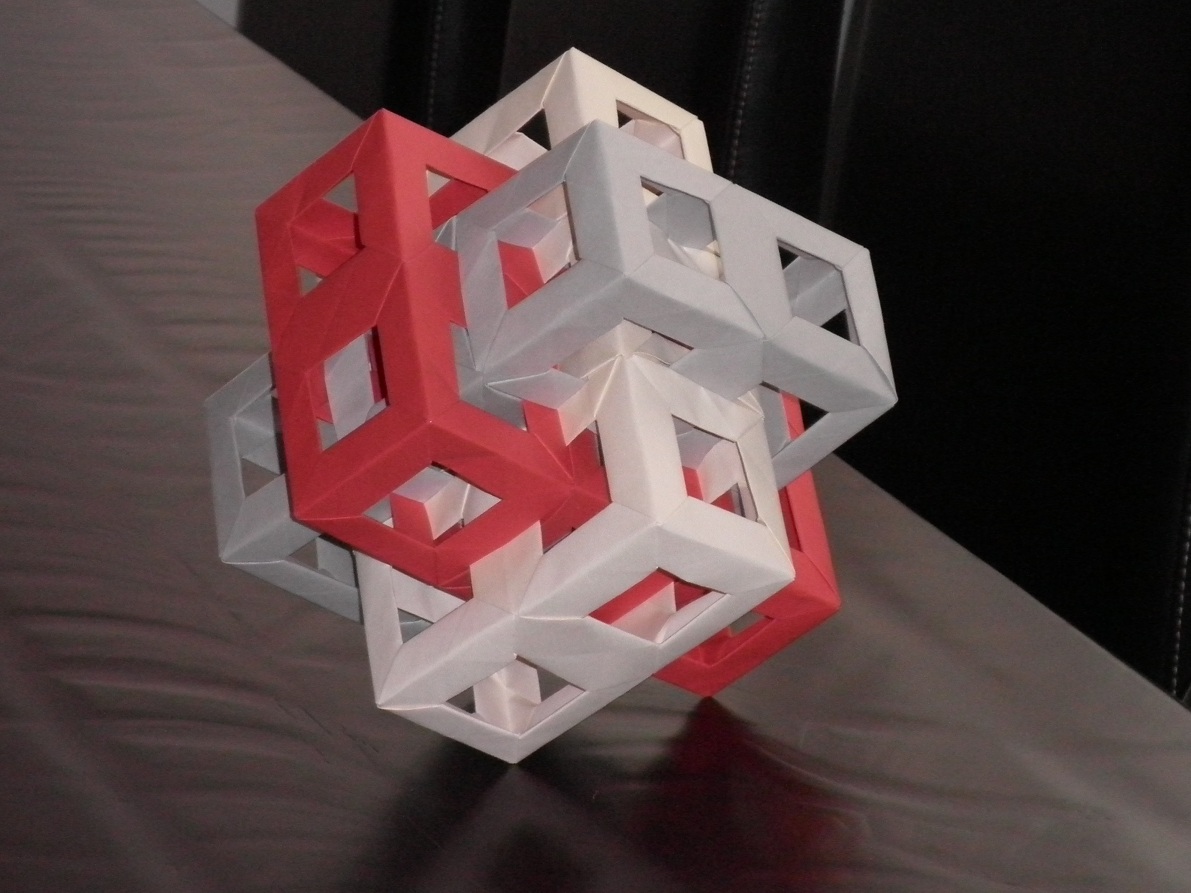
Three Intersecting Boxes
Each of the boxes is made of 56 pieces (a total of 168 pieces).
The pieces are not identical and divide into two kinds. One of the kinds
requires scissors to make (sorry).

Icosahedron (with Pyramids on the Faces)
Made of 60 identical pieces in 6 colors.
The symmetry group is A5. The coloring is just the orbits of one of
the copies of D5 in A4 (6 colors for 6 orbits).
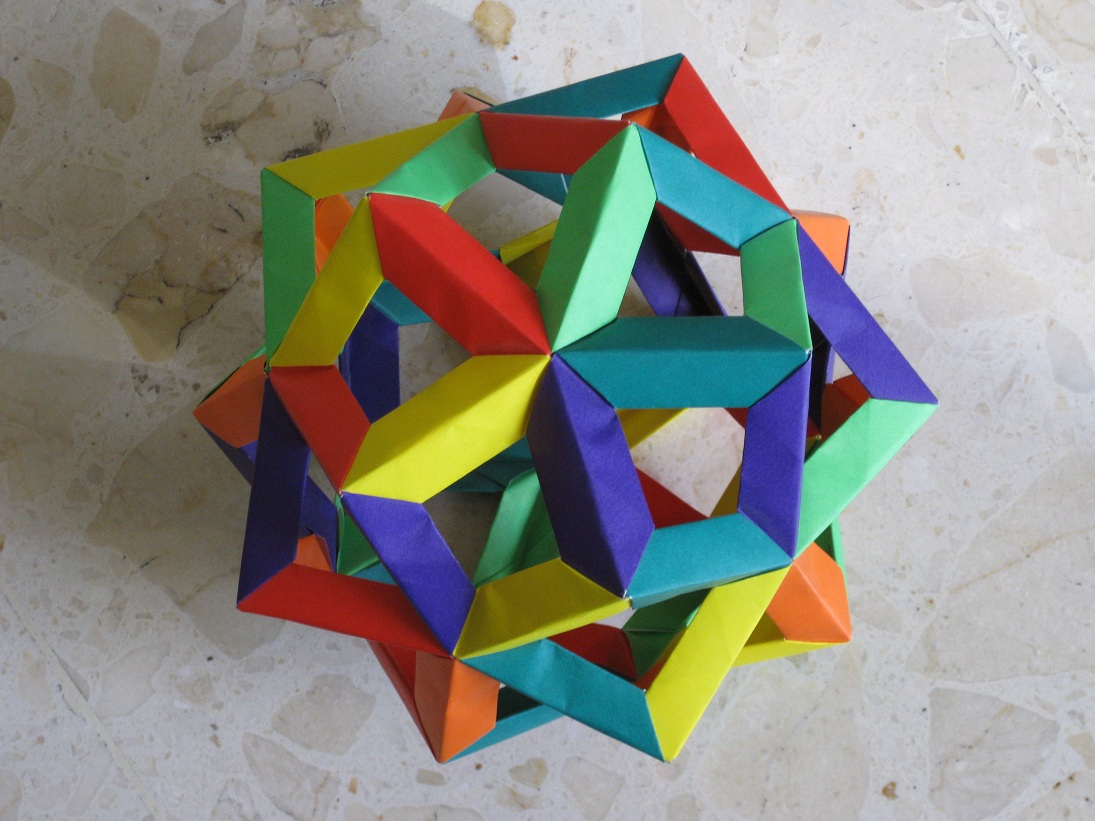
Omega Stars
Made of 6 identical pieces.
The points of the star form a root system of type A3,
or alternatively, a cuboctahedron.


Buckyball (or Truncated Icosahedron)
Made of 90 identical pieces (the so called "PHiZZ units") in 5 colors.
The symmetry group is A5 and the coloring "proves" it: Any rotation of the solid
induces a permutation of the coloring (which consists of 5 colors). This map
induces an isomorphism of the symmetry group into the group of even permutations on 5
colors.

Another Buckyball
Made of 90 identical pieces (the so called "PHiZZ units") in 2 colors.
The symmetry group of the coloring is dihedral.

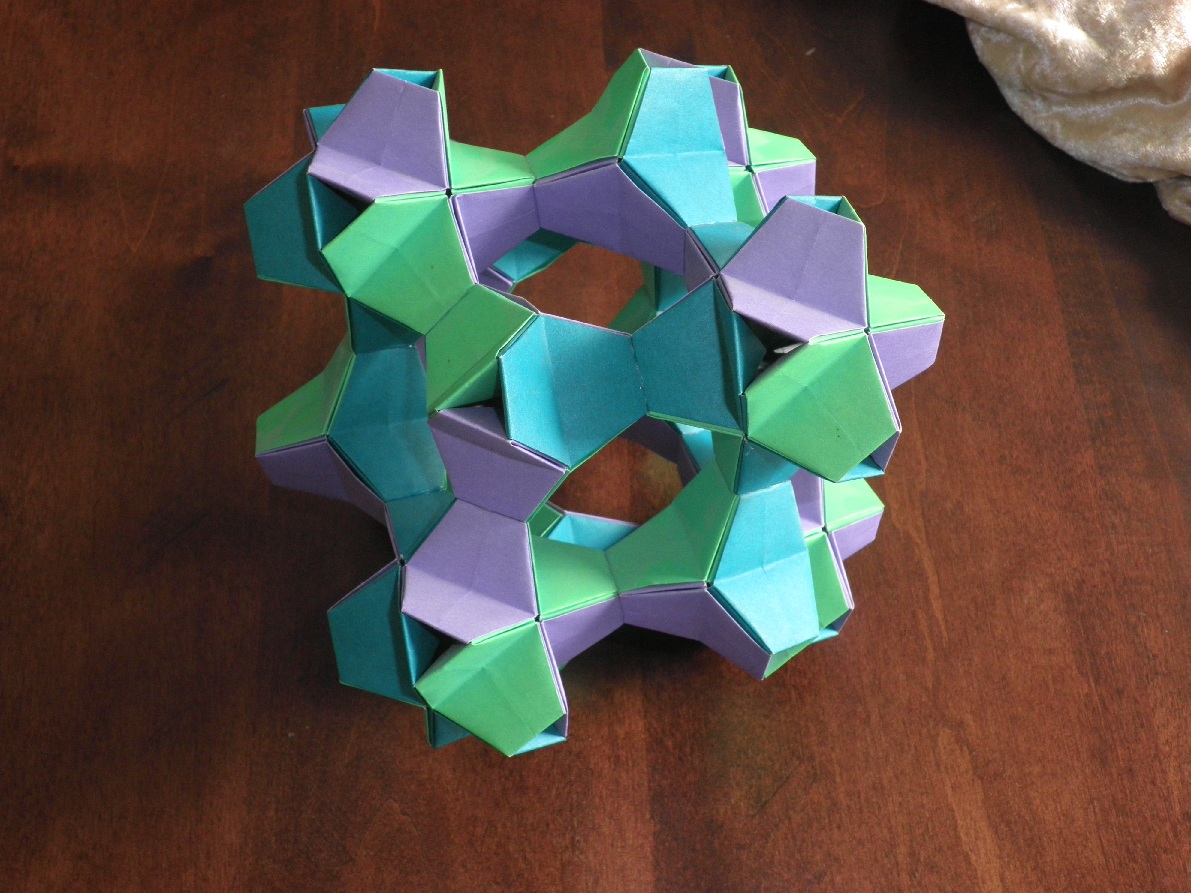
Tetrahedral-Octahedral Honeycomb
This construction is made of 6 octahedra and 8 tetrahedra (literally) glued together to
form a tetrahedral-cctahedral honeycomb.
The construction can also be understood as a rhombic dodecahedron.
Made of 120 "turtle units" in three colors.
Dodecahedron
Made of 30 identical pieces in 6 colors.
The symmetry group is A5. The coloring is just the orbits of one of
the copies of D5 in A4 (6 colors for 6 orbits).
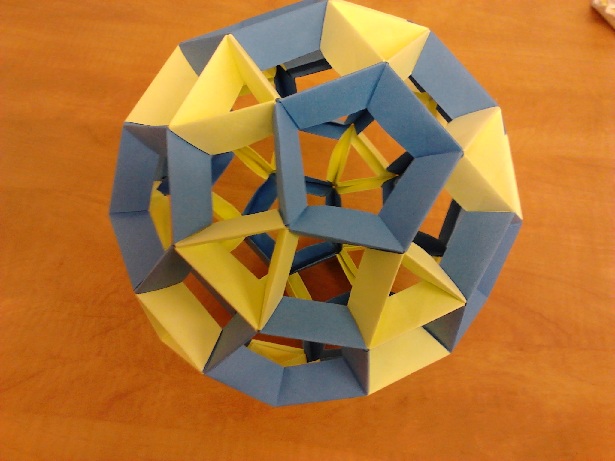
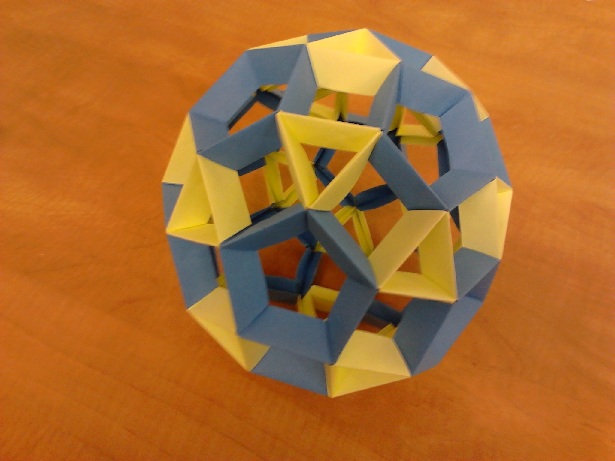
Rhombicosidodecahedron
Made of 120 identical pieces (standard 135 degrees units) in two colors.
Flowery Thing
Made of 90 identical pieces in 3 colors.
It is not easy to explain what is this solid. Its
symmetry group is A5, so it is of the same family
as the dodecahedron and icosahedron.
The coloring is unbalanced in the sense that the amount of units of each color is different.
It is designed to resemble cherry flowers.

Cuboctahedron (with Pyramids on the Faces)
Made of 24 identical pieces in 4 colors.
The symmetry group is S4 and the coloring "proves" it: Any rotation of the solid
induces a permutation of the coloring (which consists of 4 colors). This map
induces an isomorphism of the symmetry group into the group of permutations on 4
colors.

A3-tilde Coxeter Cells
Each of these pyramids is made of 2 rhombic units (by Nick Robinson) of different orientation.
The tetrahedron obtained in this manner is a basic cell of the euclidean coxeter
complex of type A3-tidle.
As a result, one can tessellate a 3-dimensional euclidean sapce with it. Moreover, it turns
out that eight A3-tidle cells can tessellate an A3-tidle cell twice their size (see the corresponding picture).



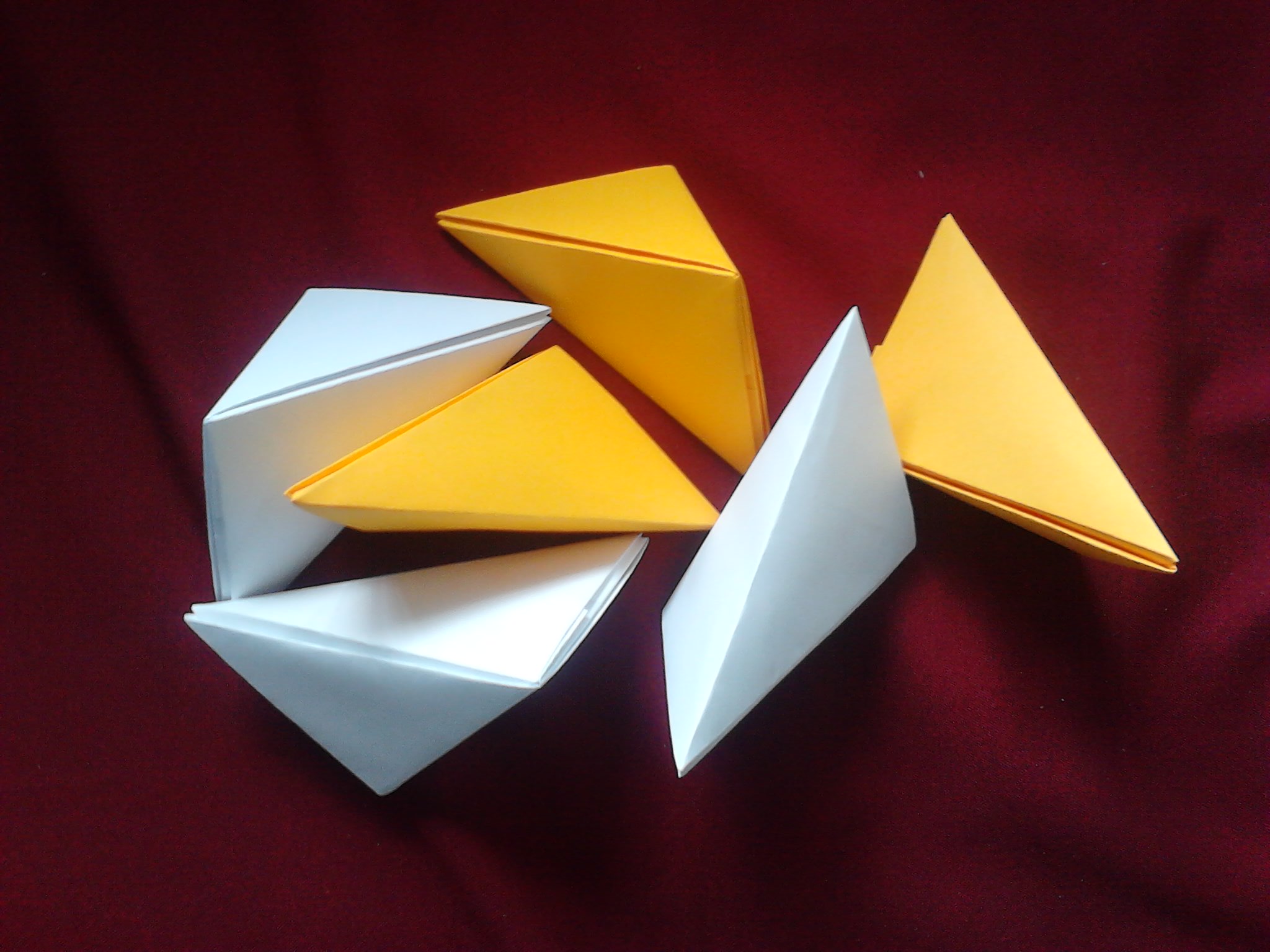
C3-tilde Coxeter Cells
Each tetrahedron is made of 2 non-identical units of seld-design. The orange and the white constructs have reversed orientations.
The tetrahedron forms a basic cell of the euclidean coxeter
complex of type C3-tidle.
As a result, one can tessellate a 3-dimensional euclidean sapce with it. As with
the A3-tilde cells, eight C3-tilde cells can
tessellate a twice bigger C3-tidle cell. One needs 6 cells of a given orientation and 2 cells
of reversed orientation to do this.



Snub Cubes
Each of the snub-cubes is made of 60 identical pieces. The right snub-cube contains an "omega star" made of additional 6 pieces.
The symmetry group is S4.
For more information about snub-cubes, see here.

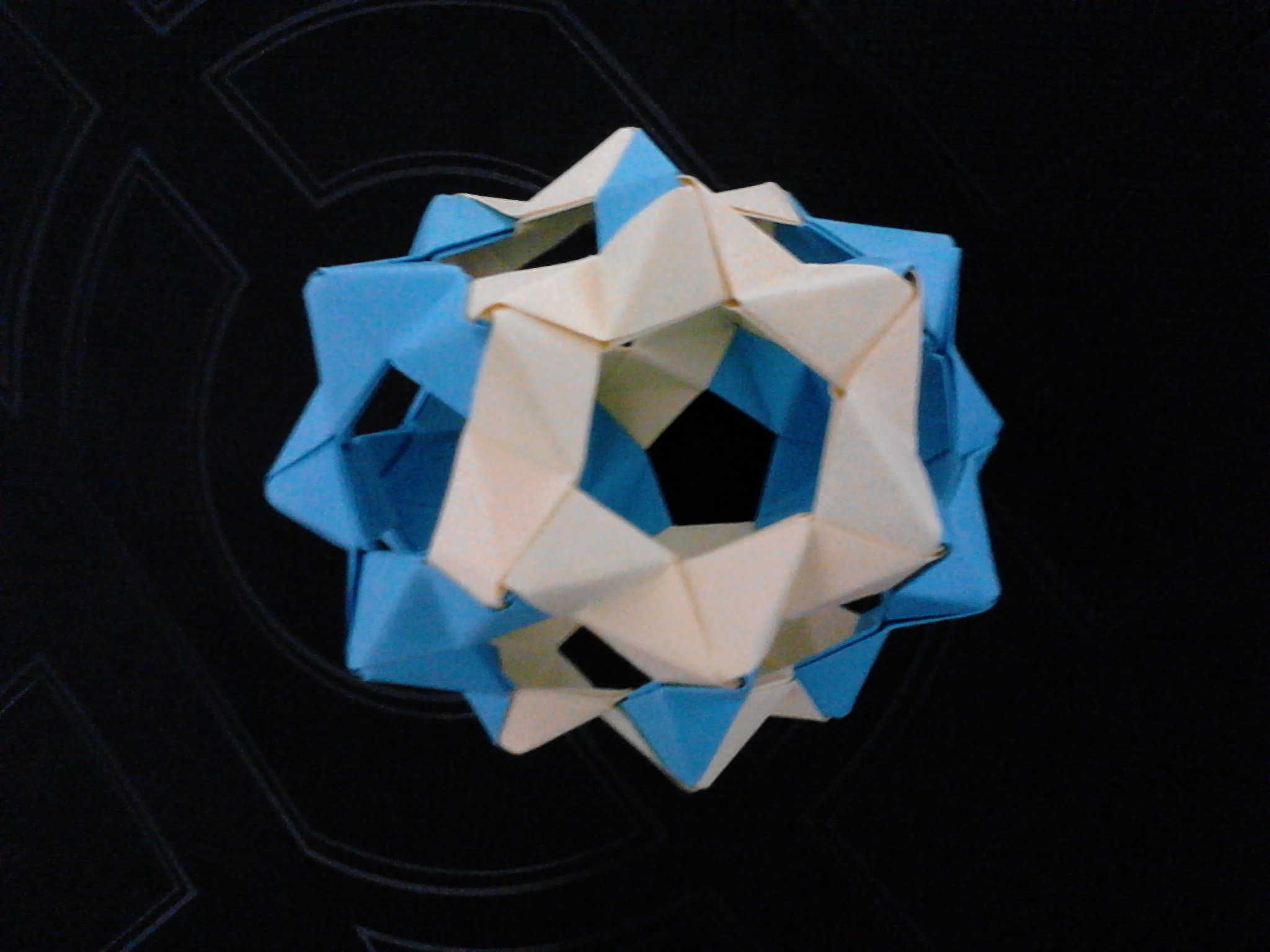
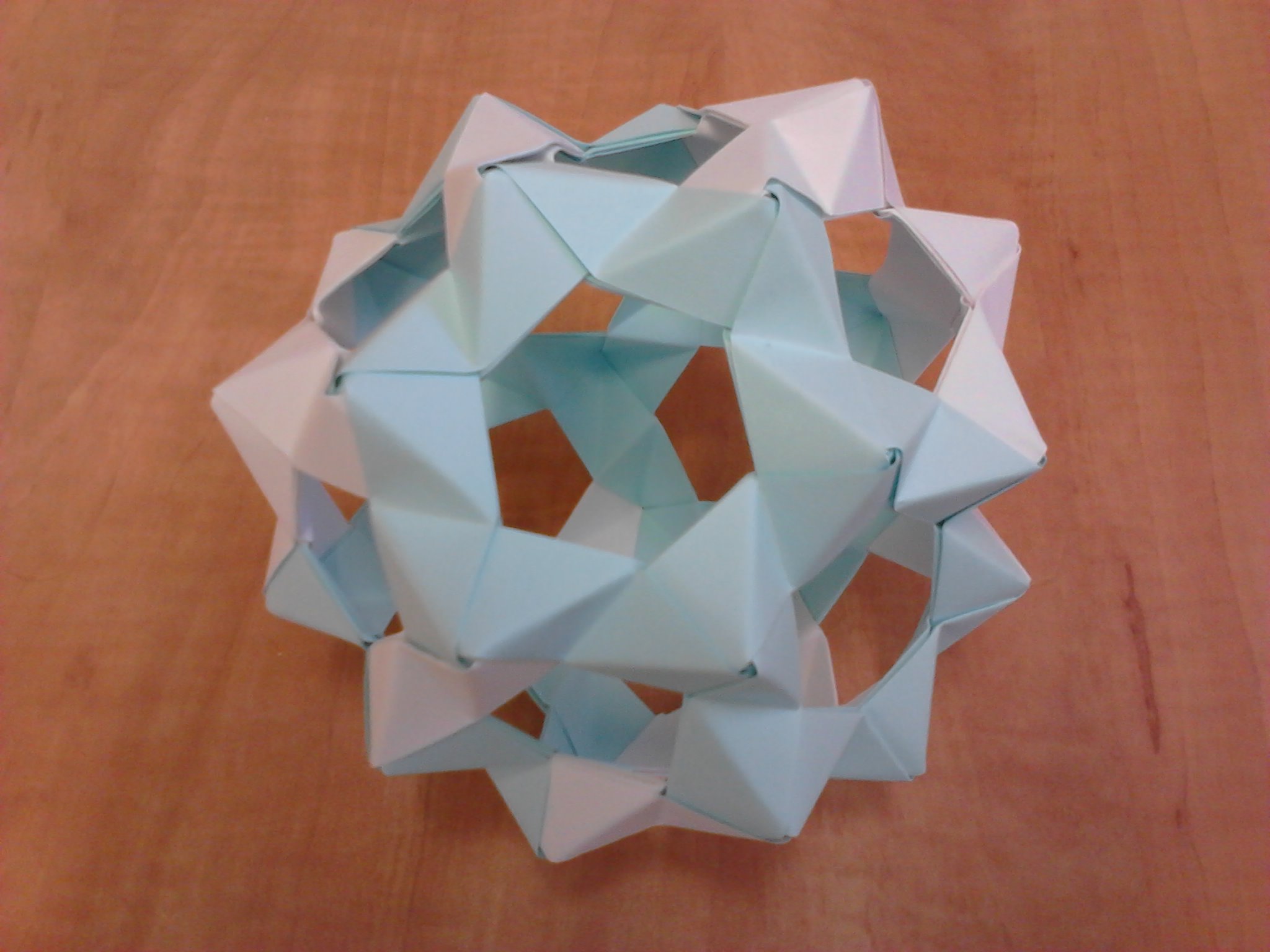
Fullerenes
A fullerene is a solid made of (possibly non-convex) hexagons and pentagons which is homemorphic to a shpere and such that three faces meet at every vertex. This forces the number of pentagons to be precisely 12. The dodecahedron and the buckyballs above are fullerenes with 0 and 20 hexagonal faces, respectively. Below are fullerenes with 3 to 5 hexagonal faces.



Small and Great Stellated Dodecahedra
Each polyhedra is made of 30 identical pieces.
These polyhedra are two out of the four regular star polyhedra (also called Kepler–Poinsot polyhedra),
see here for more details.
Constructed on 2014 at HUJI, Jerusalem.
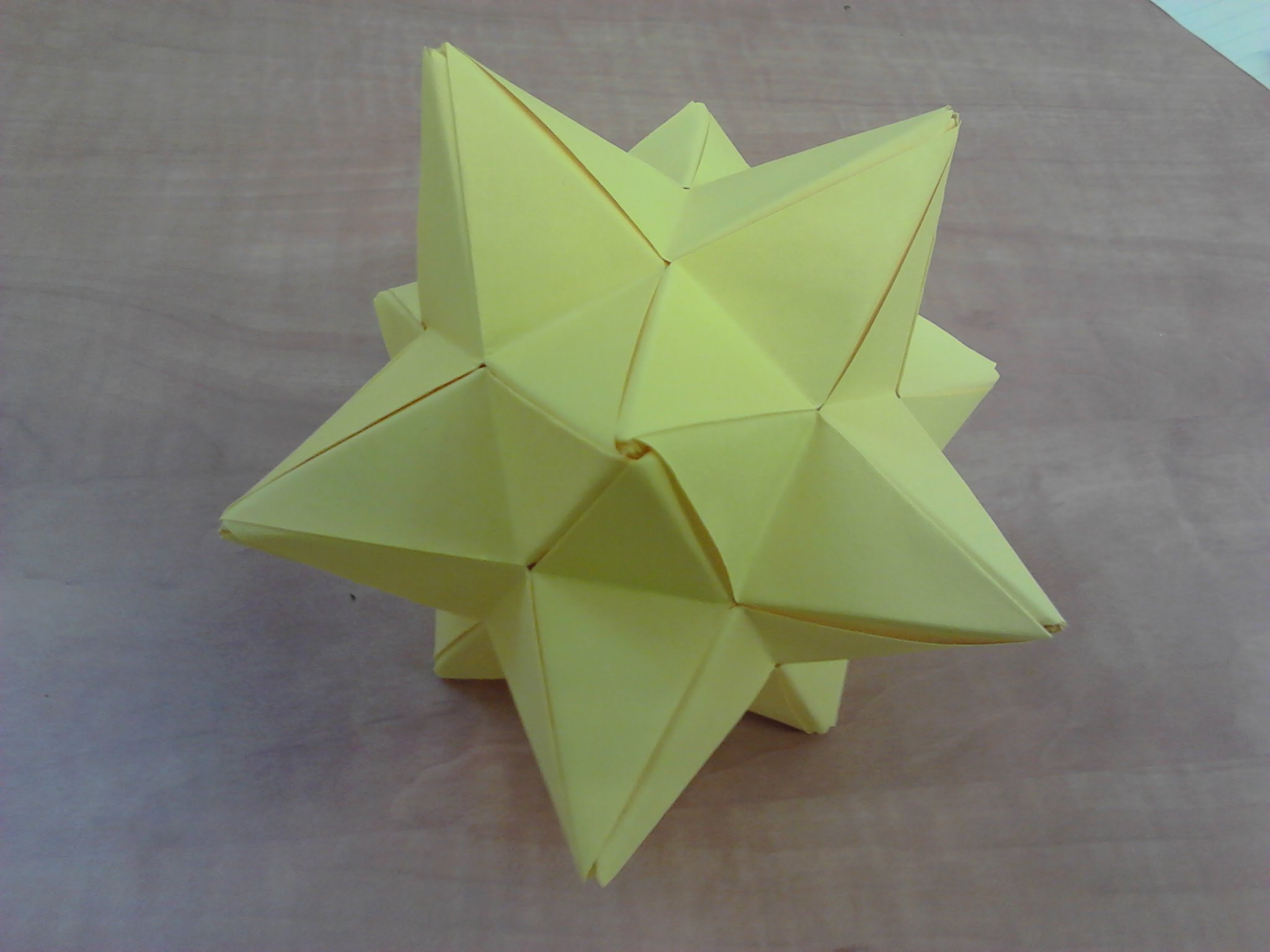
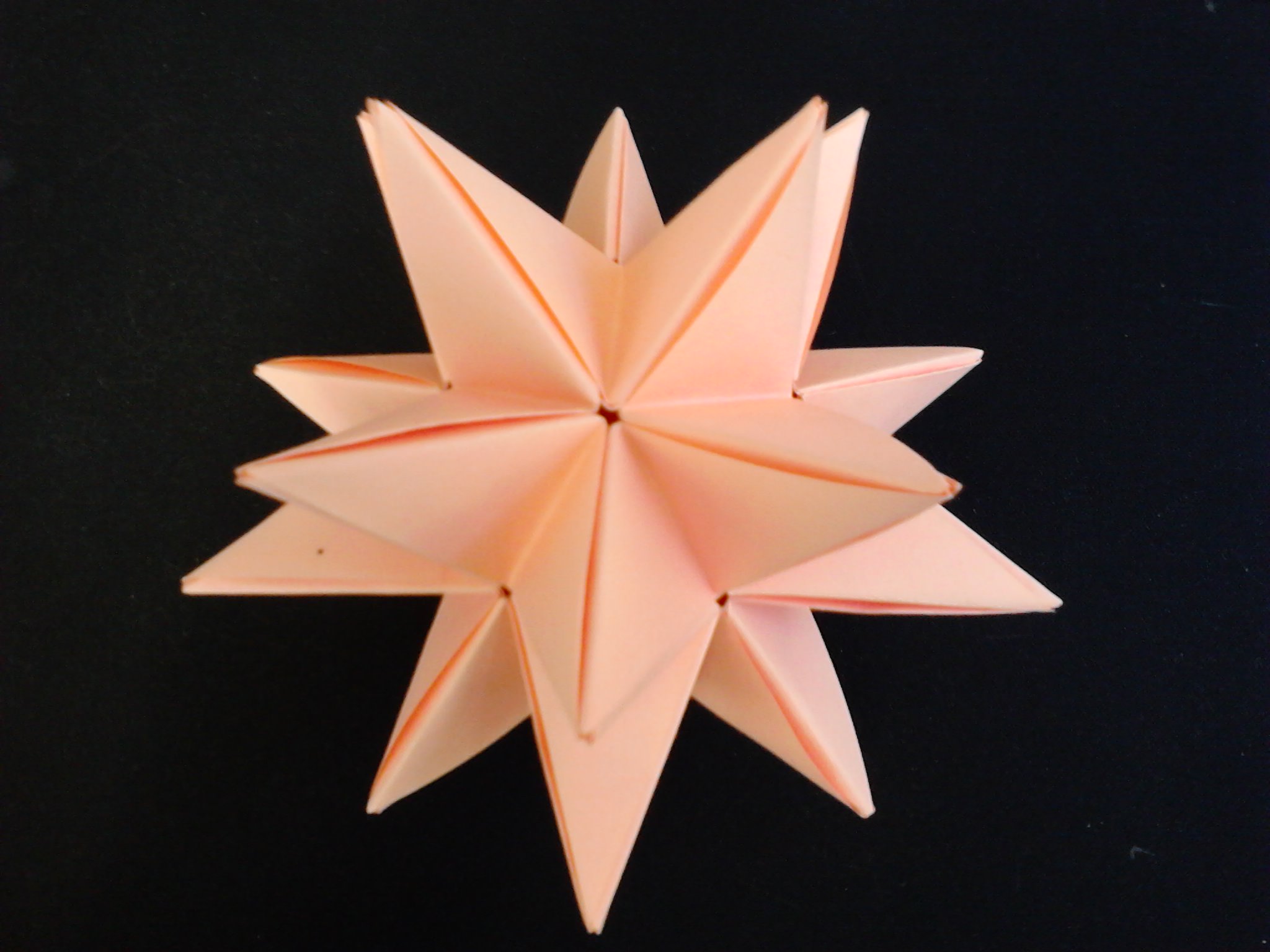

Blintz Icosahedron
The model is called "Blintz Icosahedron". It is not an "honest" icosahedron, but rather a tesselation
of six planes with the same symmetry group.
Made of 30 identical pieces in 6 colors.
Constructed on 2013 in Katamon, Jerusalem.

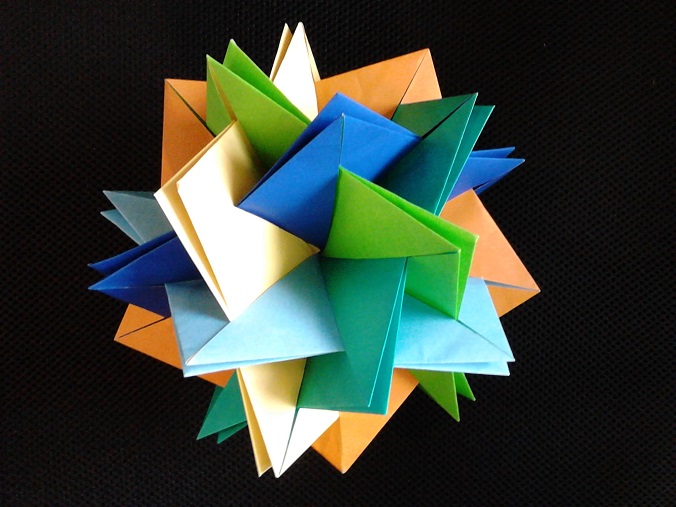
Icosahedron (with Truncated Pyramids on the Faces)
Made of 30 identical pieces ("turtle unit") in 5 colors.
Constructed on 2014 in EPFL, Lausanne, Switzerland.

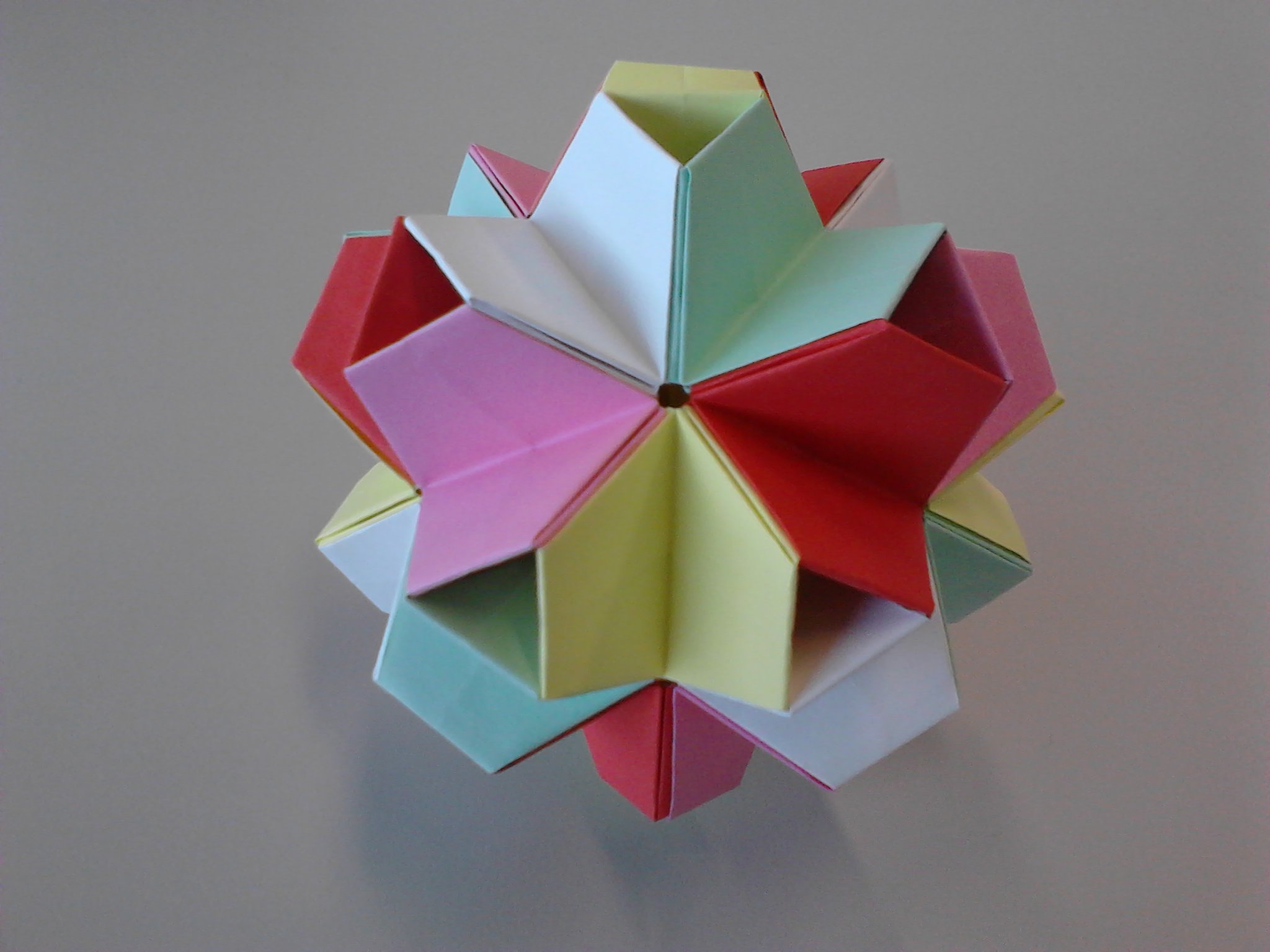
A Compound of Five Octahedra
Made of 30 identical pieces in 5 colors.
Constructed on 2015 in Netanya, Israel.

Fullerene with 30 Hexagons and 80 Vertices ("C80")
Made of 120 identical pieces in 5 colors.
Constructed on 2015 in Vancouver, Canada.


Icosidodecahedron
Made of 60 identical pieces in 6 colors.
Constructed in Vancouver, 2016.